Every once in a while I stumble across Piaget’s 7 Conservation Tasks and then I move on. This week was different because my thoughts around conservation won’t go away.
In a recent meeting with kindergarten and 1st grade teachers we discussed the importance of students being able to decompose and unitize number. This meeting has left me thinking that conservation is an conceptual underpinning of both skills. But I could be wrong.
In an effort to explore conservation we shared some videos in K-1 classrooms around our district.
We started here…
We paused the video at what do you notice and we shared our thoughts as a class.

Gotta love what Kindergarten students notice.
Right before the flattened clay was placed back on the scale we hit pause again…

Students predicted if the clay would weigh more, less, or the same using a slip of paper and unifix cubes (below). With this being a kindergarten class we spent time talking about letter sounds at the beginning of each word and what each word meant.
Some students struggled with the letters/sounds but the majority of students were able to identify where more, less, and the same were located. This turned out to be a quick and efficient way to formatively assess in multiple areas.
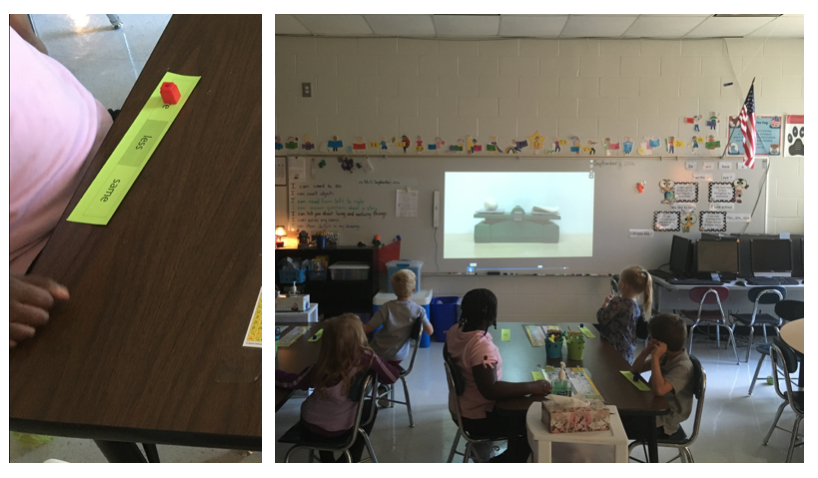
We gave students an opportunity to share and explain their predictions. Sharing predictions was the easy part. Explaining and justifying our predictions, not so much.
So we played some more and followed up with this…
And this…
And finally this one…
The whole idea of unitizing is a foundational understanding that students should own by the time they leave the primary grades. It’s our hope that by introducing the concept of conservation as it relates to length, weight, and liquid, that students are able to make connections across different contexts.
We hope that these contextual connections begin to help students explore how 1 ten is the same as 10 ones.
But it’s the fourth week of school and we know we have our work cut out for us.

Graham – I like the videos and think it’s always good to get students asking questions and making observations. This will help with problem solving and hopefully decrease the number of students who do not have an entry point into problems and simply get stuck with no way of starting or restarting. Are you using an iPad to shoot these videos? Tripod?
I don’t see what the ten ones is one ten thing has got to do with area (a very difficult concept). One might as well use length, or even volume, as far as the bricks are concerned.
The cubes have aspects of length, area, and volume. When they are linked up in a line, their length aspect is accentuated. When they are unlinked and spread across the surface of the table (two dimensions), you could argue that their area aspect is now highlighted
Cool stuff, Graham. Now, prepare for a bit of a ramble. Buckle up and I hope you stay with me to the end!
It gets me wondering(!) how the Notice/Wonder routine impacts the experience for both students and teachers vs. the original tasks.
The originals were meant for assessment and the closed nature of the questioning was targeted to assess very specific conceptual understanding. The tasks were not aimed at constructing student understanding about conservation.
Notice/Wonder-fying them definitely changes this assessment:construction (im)balance. Here, we’re trying to do a bit of both, so I’m wondering how effective you are finding these tasks in terms of both assessment and construction? Particularly over time?
Weight task aside (the scale offers the opportunity to predict, giving students the opportunity to essentially answer the Piagetian assessment question), the essential questions are not built in. Where did you go from the Notice/Wonder prompt? Was it a goal to steer each task to generate and explore the essential question? If so, we would get some definitive answers as to students’ possession of conservation. If not, I’m not so sure we would?
Now, assuming we generate the essential question (Are the sticks still the same length, or is one longer?), we would likely then explore it and come to a concrete conclusion. In the process, some students begin constructing conservation of length.
However, can we still use the Length task to assess conservation in the future? Students already know the answer, whether they’ve actually acquired conservation or not. Or, are we equating the ability to recall the result of the task when the same situation comes up in the future as equivalent to acquisition? Is this similar or different to the young child who “knows” 2 and 2 is 4, but if you held up 2 and 2 fingers, they would need to count them all. Hmm…
I think this is on my mind because at Carroll, we have a Piagetian Cognitive Development program that assess kids using the conservation of number and mass tasks. We had a student, who, over many assessments did not show acquisition of conservation of mass, until one time he said, “Oh, I know this – they’re both the same. My dad told me that they’re always the same.” Great, now he “knew” it, but had he really acquired it?
So, I think what I’m getting at is, we’ve only got a limited number of assessment opportunities (if we care about that piece) once we start using these tasks as explorations (7 at most, and probably fewer, since I think volume is significantly more cognitively complex than the others), so we might want to be deliberate in when we use them if at some point we want to be able to definitively know if a student has acquired conservation.
Finally, I like your connection to unitizing, and it’s interesting how the 1 ten, 10 ones task is essentially Piaget’s conservation of area task – did you have this connection in mind as you were doing it?
I think it depends on how we plan to use the information we gather for the assessment. Are we looking at it as formative or summative assessment? If it’s summative then we can only use it once but I think we could show the video without the reveal and use it multiple times with students and see how/if their response changes over the course of a year.
Agree 100% here but I’m thinking that when the little man says he “gets it” I would replicate the tasks but change the conservation part by adding to OR taking away from one so that they’re not equal. Does that little man still say they are the same? Probably, if there is limited to no understanding. I really appreciate you sharing your thoughts here Peter. I think it stresses the importance of understanding why and when we should assess.
And in terms of the area connection, when I was looking at the tasks I struggled to create the area model so I appreciate you sharing that I actually did it. Nice catch and thanks for pointing that out.