Before I travelled to NCTM I was finishing up some district PL with K-1 teachers. As part of the workshop, teachers were asked to engage in a Number Sense Trajectory Cut-N-Sort
- match header and descriptor
- place in the order that students learn number
Easy enough right?
Of all the times I’ve used this activity only a handful of teachers have correctly ordered the trajectory. FWIW-I had no idea number sense started with subitizing and comparison either.
Personally, it’s a favorite activity because it helps identify teacher misconceptions, which in turn helps move students through the learning progression. Even though it shines a light on misconceptions, teachers really like it and that has to count for something.
After teachers completed their poster we shared and discussed using the K-1 Learning Trajectory document below.
Let me be clear… this is by no means my work.
I created this document to be included in the Georgia Frameworks 5 years ago. This work is a mash-up from 3 of the most respected people in K-2 mathematics and they are educators that have drastically influenced the teacher I am today; John Van de Walle, Doug Clements, and Julie Sarama (who I just realized are on twitter…score!).
I shared this with some teachers after Christina’s session at NCTM. She did an amazing job explaining the importance of number relationships as mentioned by Van de Walle. Since then I’ve received some emails asking me to share this trajectory, so I figured I’d share here as well.
Be sure to include this book on your summer reading list. It changed the way I interact with students in the primary grades and I keep referring back to it 5 years later. Thanks Doug and Julie for making us all smarter!



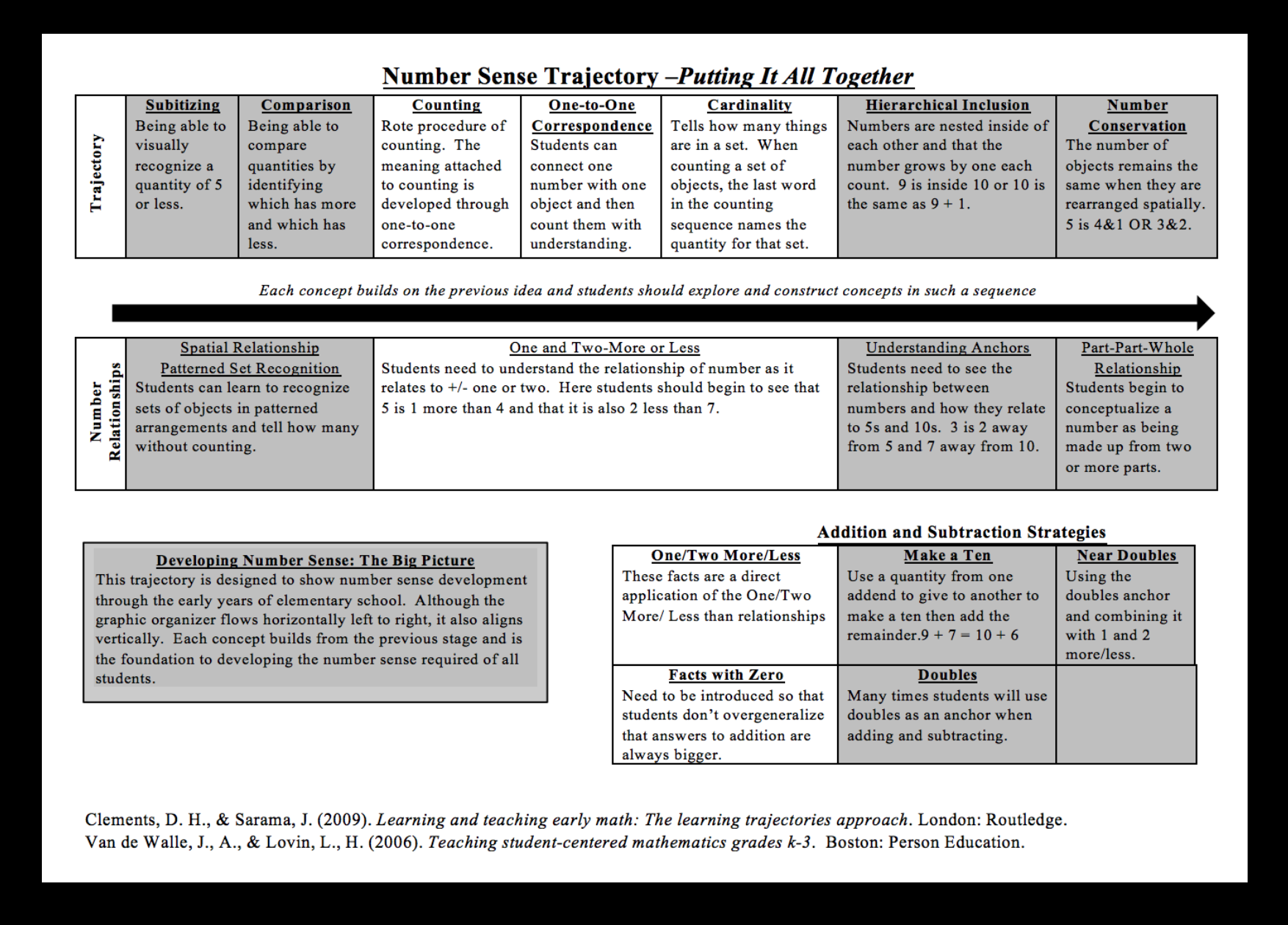

Just wondering why subitizing is listed first (on the learning trajectory). While humans might have the capacity to learn to match number words with a quantity of items, but I think the normal progression is for families to rehearse actual counting. Comparing (without numbers) amounts seems inherent to human development, so that seems logical that it appears early in development. Subitizing seems to necessitate concerted practice – 4 dots equals four – which most families don’t really get into. I can recall being able to count to 100 (probably with a few errors) at the start of kindergarten. I probably didn’t pick up knowing the dots til 1st or 2nd grade. Again, that was my experience; I could perhaps have done such earlier, just wasn’t exposed to it.
Thanks for the comment Dan and I can definitely relate to what you’re saying here. I think parents unknowingly support an understanding of subitizing before they get to school, not just the subitizing we’re used to.
There are 2 types of subitizing (conceptual and perceptual). Most of us are familiar with the understanding of conceptual subitizing however the majority of students have exposure to perceptual subitizing before ever reaching Kindergarten. Think of it this way…when a student is told how to hold their fingers to show their age of 3, do they have any idea what those 3 fingers actually mean? I would say “no”, but they can tell you what the name for the number of fingers they are showing. Same goes for the pips on dice. Kids can tell you how many pips after some exposure but only because they are able to recognize and recall the name for that particular arrangement of pips.
Doug Clements and Julie Sarama have a great article on the different types of subitizing that you might appreciate. http://gse.buffalo.edu/fas/clements/files/Subitizing.pdf
Cheers,
Graham
Hi Graham
I am following all your work and activities. I do have a question based on your trajectory summary. My understanding is that kiddies may not learn these in a specific sequence (not linear). Am I incorrect or is it more linear as you suggest?
Thanks,
Debbie
Hi Debbie,
My understanding is that students work through this progression as if it’s linear although some students may skip stages. I This work is heavily influenced by the work of Doug Clements and Julie Sarama. If you’re not familiar with their work you definitely want to check it out. Great stuff!
Thanks! Will put the book on my list to read. I love a good math book!
I think the cut-n-sort cards are great. I put the book on my Amazon wishlist!
Thank you for the reminder to go back and read Doug Clements’ and Julie Sarama’s book. In the past, I have had teachers work with the ideas–definitions and examples of these big concepts–but never did it as a card sort and poster. Appreciate the idea–and if you don’t mind, will share it with some groups over the summer.
Absolutely! Please share as you see fit (it’s kinda the reason I posted it).
It was an amazing shift for me and it’s been such an eye opener for teachers when they see that children can subitize without counting and cardinality. But then that also bring to light the difference between the conceptual and perceptual subitizer.
Please share how it goes!