It’s our fault. We have no one to blame but ourselves.
We unknowing pigeonhole student thinking with the manipulatives we use. Take fraction tiles for example. Much to my disappointment, they come with labels and it kills me.

Manipulatives that come pre-labelled ruin everything I want from a lesson. Sure you can flip them over but the label on the backside keeps rearing its ugly face and traps lots of student thinking.
Sure there’s Cuisneaire Rods but most teachers don’t have $200 to fork out for a class set. But I think it’s fair to say that most teachers would fork out $4 for some fine steel wool.
Presto! Fraction-Cuisen-Part-Whole-Tiles!
As I finish up planning for my Grassroots Workshop in Anaheim next month, I can’t help but think how faceless manipulatives help us guide students through the progression of learning because of how they can be flexibly used.
When we label items we avoid lots of opportunities to listen and build on student intuition. This was something I took away from Tracy’s most recent post. Tracy helped me see that I need to provide students with more opportunities to play and explore…WITHOUT INTERFERING.
I think this gives them a much better chance.
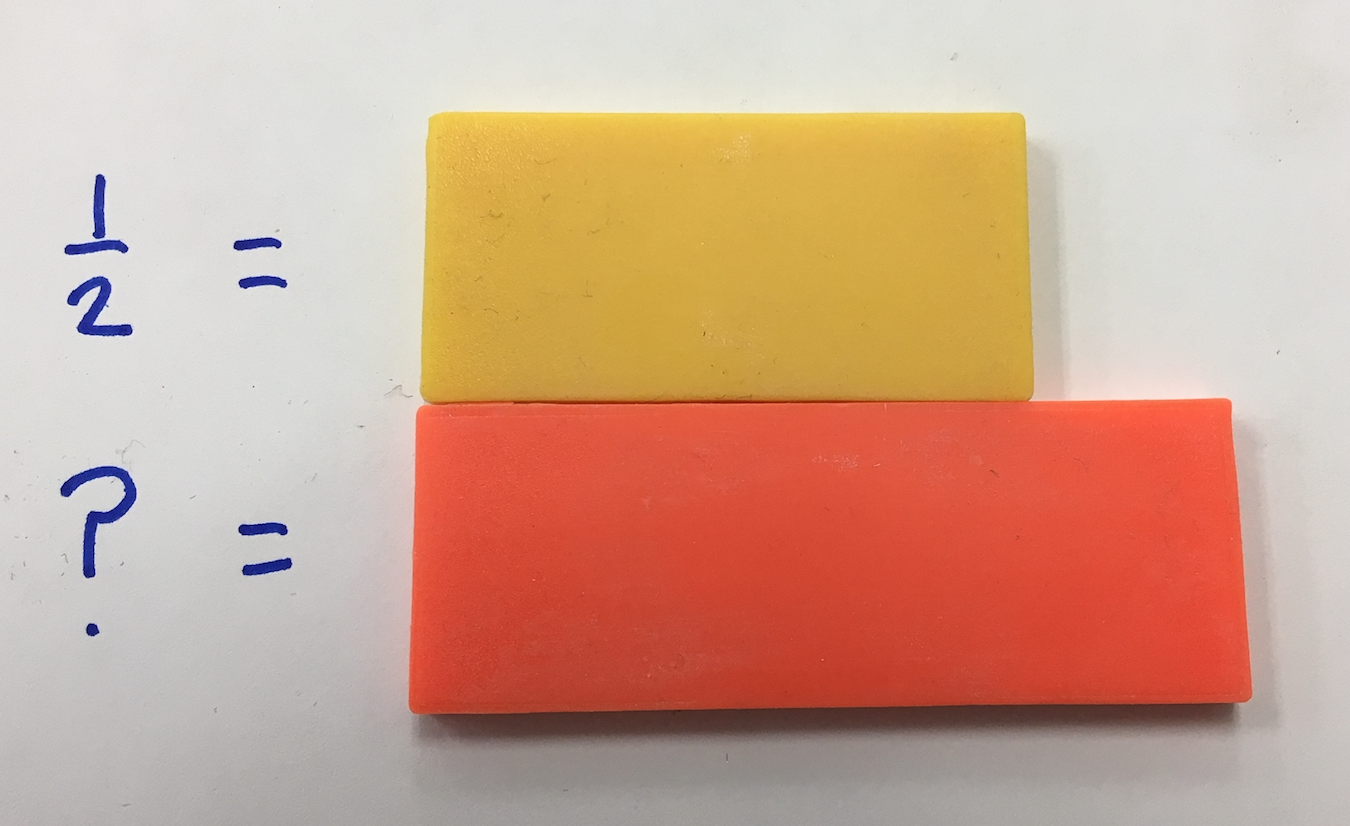
What the value of the orange? It sure isn’t a third.
With that being said, even when we do get our hands on unlabelled manipulatives we usually assign the same value to each piece…every time.
Pattern blocks are a perfect example. Most of the time we assign the hexagon a value of a whole. This creates a false sense of understanding which is really hard to unmask.
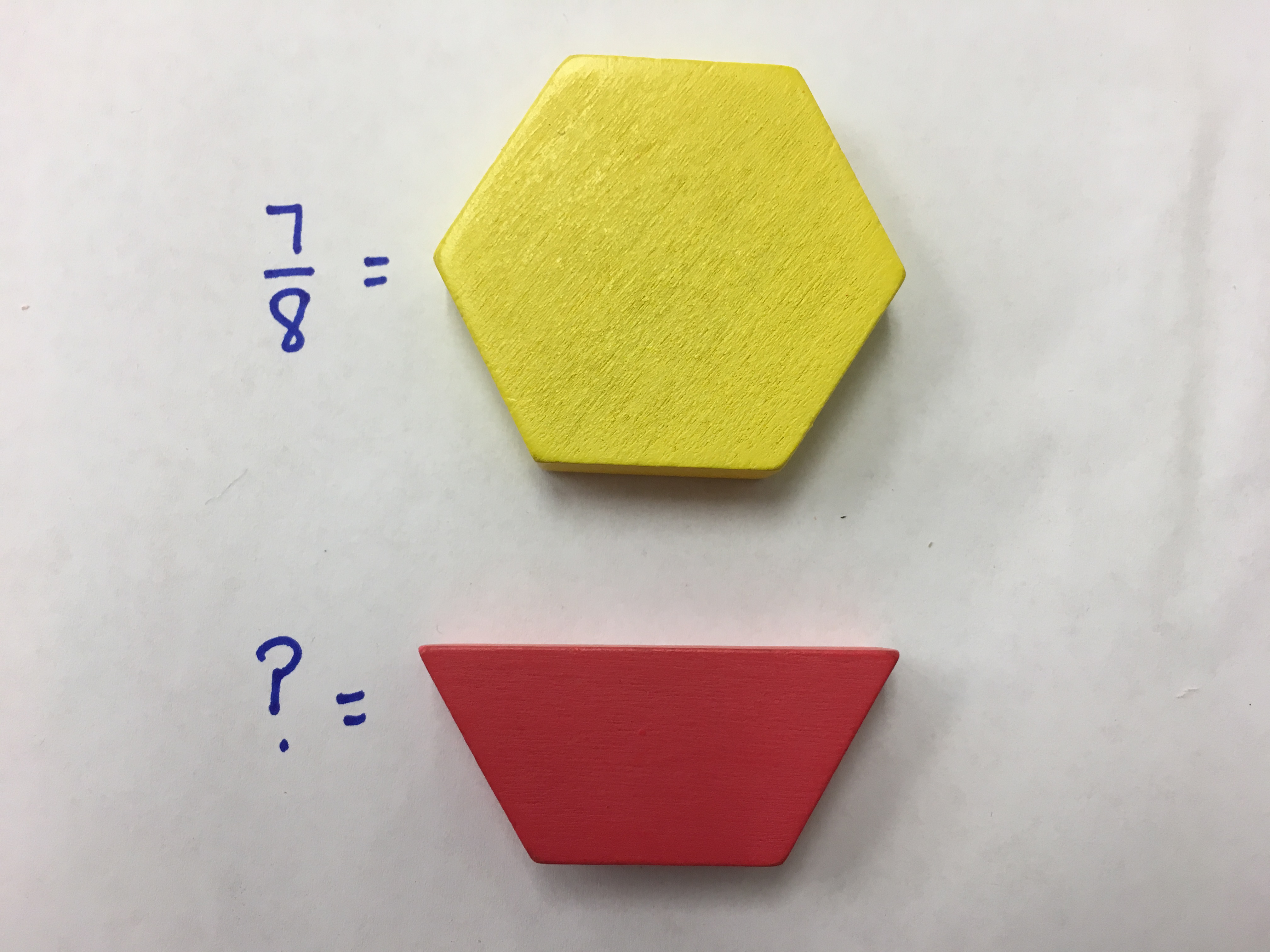
Where’s my head at right now?
I’m continually seeking ways to undo student learning and identify what understanding they truly own. In order to do that, I need to be sure I’m not “pigeon-wholing” student thinking.
Question: Where else in mathematics do we pigeonhole student thinking? This can be within our instruction OR through the use of manipulatives.
Please share your thoughts below.

Genius! We need to really think and be purposeful what scaffolds we provide to students and how those scaffolds may be helping or limiting overall student mathematical understanding.
I agree that we need to use multiple representations of a concept before students are able to generalise. For example in early maths learning we might use multiple representations for place value: bundles of popsticks, tens frames, strings of ten beads, MAB. But unless students get the opportunity to use the materials to model the concepts for themselves, unless they get to appropriate the structure and develop a need for structure, these tools run the risk of becoming another form of algorithm to be learned and sometimes misremembered: “which one means ten again? The short or the long?”
BTW: a cheap, easily accessible version of Cuisenaire rods can be found on iPad. It’s an app called Numblox. Much cheaper than a set of blocks and you don’t have to worry about losing or stepping on any pieces!
Another option is to laser-cut your own manipulatives from perspex or similar plastics. Some schools have these available and the materials are relatively cheap. If you don’t have the cutter available, many local shops might have offcuts that they can give you for the purpose.
Thank you for sharing your ideas through this blog and twitter. You are helping me to be a better math teacher, one post/tweet at a time!
No problem Mike and glad to help out.
Wondering if fingernail polish remover would take off the numbers? But I’m about to find out! Love this. I’ve been using cuisinairre rods this way, but like this idea too!
Ha! My wife said the same thing when I posted this and I keep forgetting to give it a try. I tried graffiti remover and industrial strength paint thinner but they melted the plastic. Nail polish remover didn’t exactly come to my mind for the obvious reasons.
That’s why all of us are smarter than one of us. I’ll give it a whirl and report back. Thanks Heidi.
Why I never thought to grab some steel wool… I guess I’ll never know! Great idea, Graham. The best part about this – as you mentioned in your post, is that if teachers already have these, they now have a set of Cuisenaire knockoffs. And now students will need to reason about the quantities rather than just read a fraction printed on a tile.
As for Joe’s question above, my answer is yes! I’m not a big fan of base 10 blocks for a host of reasons. “Pigeon holing” by forcing our understanding on students with labels of 1, 10 and 100 to the “unit cube,” rod, and flat has the same result with students as the labels on the fraction bars. The only difference is that there is nothing written on the blocks. Over time, students become parrots of our understanding. Example –
Teacher: And what does this rod represent?
Students (often in chorus): Ten
The question that’s rarely asked and answered… How do you know? When I’ve asked it, the response has been something like, “My teacher told me last year.” Students don’t really know it and they can’t explain it. So, I guess when students parrot, we know we’ve pigeon holed. That should be ill-eagle (sorry… had to throw that in).
One of the most important ideas in teaching and learning base ten is that any place is 10 times greater than the place to its right. Maybe we should focus on that.
Pigeon holing! Fantastic name for this! Well done, Graham.
Cheers Mike and these are all great points you bring up. Thanks for sharing. I definitely agree that the concept of 10 times greater really gets lost when we use base-10 blocks. It relates to the activity you introduced me to from Van de Walle, the Decimal Names the Whole. There’s some pretty great understanding that needs to take place right there.
As for your “ill-eagle” comment…I have nothing else to say:-)
Nice work! You’ll be in Anaheim? I want to crash.
Agree that the pre-labeled, pre-partitioned, pre-everything takes away the opportunities for students to construct their own understanding and allow flexible use of the materials. Great hack on the fraction tiles!
I agree Graham. The labeled manips are taking learning away. Some of the tasks from the Fraction Pathway research by Cathy Bruce specifically addresses the patterning block issue and has the students change the whole using different blocks and changing the value of each block depending on the whole. There are few battles I am having in my schools to get teachers to change some of their thinking. Removing the use of fraction circles or the pizza model and getting them to use models that have more longevity like a number line, rectangular area model or pattern block is slow process but is beginning to take hold. We are fortunate our board spent a pile of money buying cuisenaire rod sets for our schools. We usually have one class set at least per school that we can move between classrooms. We are seeing their use blossom in many schools. Thanks for sharing again!
The more and more I dive into Cathy Bruce’s work and the Learning Pathways the more I discover how brilliant it all is.
I couldn’t agree more about the circle fraction pieces. I think they have a sell by date because the scalability of the tool is quite limiting in its use. Great point Mark and thanks for sharing.
I just did a blog post that that furthers shows how circles can be a difficult model for primary students. It has video of me working with one student who was having difficulty figuring out why her partitions were not turning out equal when she used that model. I think the light bulb went on for her after our little guided session.
Great stuff Mark. It definitely adds to the conversation. https://mstamp36.com/2016/12/12/being-wary-of-the-use-of-the-pizza-circle-model-for-fractions-in-primary/
First you take apart a clock, and now you deface fraction tiles. Is nothing safe? Great idea. One example that comes to mind is base 10 blocks. When fourth grade rolls around, those pieces, which have acquired very fixed meanings as 1, 10, and 100, find their meanings changed. The flat is now one whole, the longs now one-tenth, and the little cubes are now one-hundredth. Would that be an example of undoing learning? For fractions, the foam fraction circle pieces that our curriculum uses don’t have any labels on them, maybe for the reason you suggest.
Chewing on the circle fractions pieces comment Joe, but for different reasons. Wondering if the shape itself pigeonholes students thinking. But in terms of base-10 blocks, I couldn’t agree more.
Thanks for stopping by bud.
brilliant!
? = 7/16
I love that steel wool hack!
Excellent. Using pattern blocks, e.g., where the relationship between the blocks is “obvious,” but the given measure of one of them is ‘inconvenient” and students have to combine the obvious relationship with the pain-in-the-rear measurement is great. Think about what this can do for getting away from multiplication-IS-repeated-addition in the context of rational numbers. Love it.
Thanks Michael. The pre-labeling of tools has always been a bone of contention for me.
I really like the connection you’ve shared as well, about using these as a whole number tool and not just one for fractions. I think we all win when we move students from additive to multiplicative thinking. Just don’t think it’s the goal of many. Wondering why not.
Have you plumbed the depths of Keith Devlin’s several columns in DEVLIN’S ANGLE about multiplication is NOT just repeated addition, starting with “It’s Ain’t No Repeated Addition?” ? If not, prepare for a wild ride. I blogged about this issue several times, first in opposition to Devlin’s view, then later in support of it. There is no doubt in my mind that we do grave disservice to students if we leave them with the impression that “multiplication IS repeated addition” (MIRA). I am one of the founders of “MIRA-Busters,” a group dedicated to bursting the myth that multiplication is (nothing more than) repeated addition. And if you extend that to “Exponentiation is (nothing more than) repeated multiplication” and beyond, you realize that while it’s lovely that we can solve certain calculations in the integers or perhaps rational numbers by reducing EVERYTHING to addition, that works rather badly when you get to irrational numbers, complex numbers, etc. 3Blue1Brown’s videos on things like e^(pi*i) make clear the limitations of such “integer-bound” thinking.