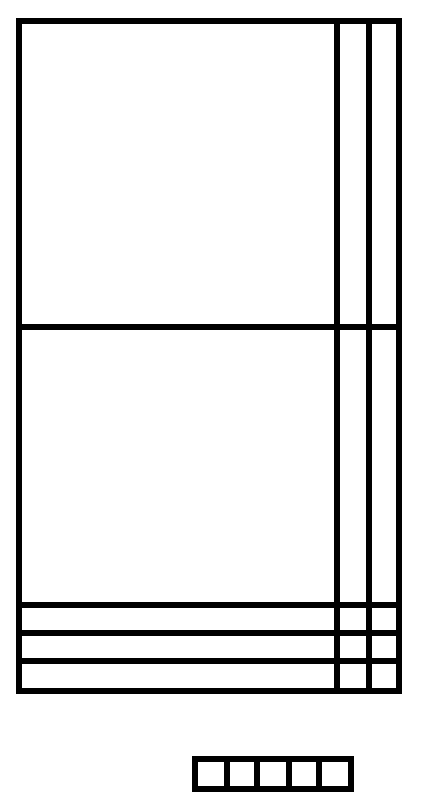
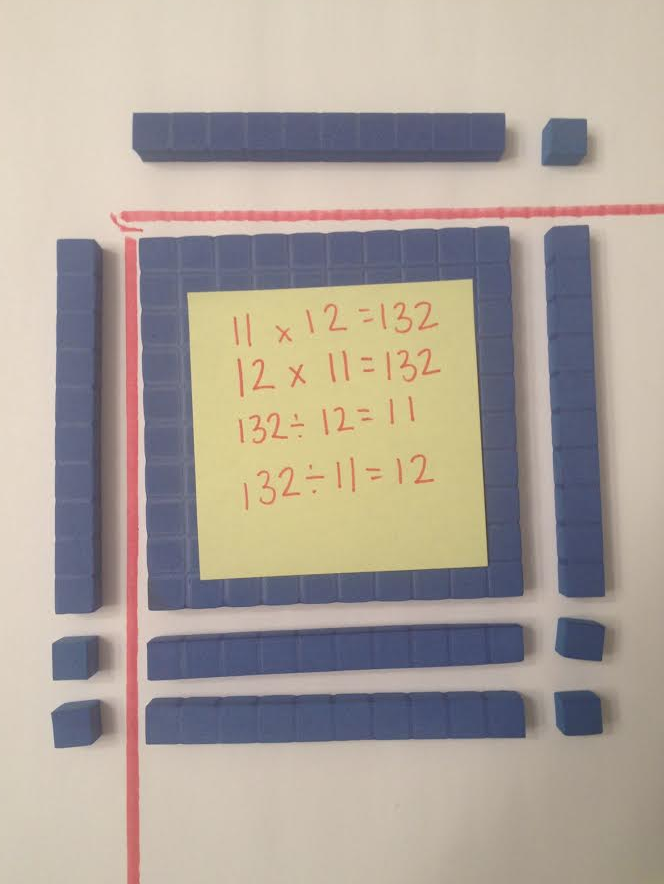
Students can create models that represent their understanding, but can they undo models that explain the reasoning of others? It was time to find out. I showed this picture to a 5th grade class and asked them to write an equation or expression that matched the area model…
Every student in the class said it was modeling multiplication however no student could account for the 5 squares at the bottom.
Why did this happen?
My takeaways: In order for students to understand what’s happening, there’s a progression of understanding that begins all the way back in 2nd grade. This understanding is built through exploration. These explorations happen in and out of context, with and without manipulatives and representations, but most importantly…it happens over time.
2nd Grade: students are expected to partition a square in to 5 rows and 5 columns. They should also use additive equations that match the model.
- 2.OA.4 – Use addition to find the total number of objects arranged in rectangular arrays with up to 5 rows and up to 5 columns
3rd Grade: students begin to model multiplication AND division with arrays at the same time to develop relational thinking.
- 3.OA.1 – Interpret products of whole numbers
- 3.OA.2 – Interpret whole-number quotients of whole numbers
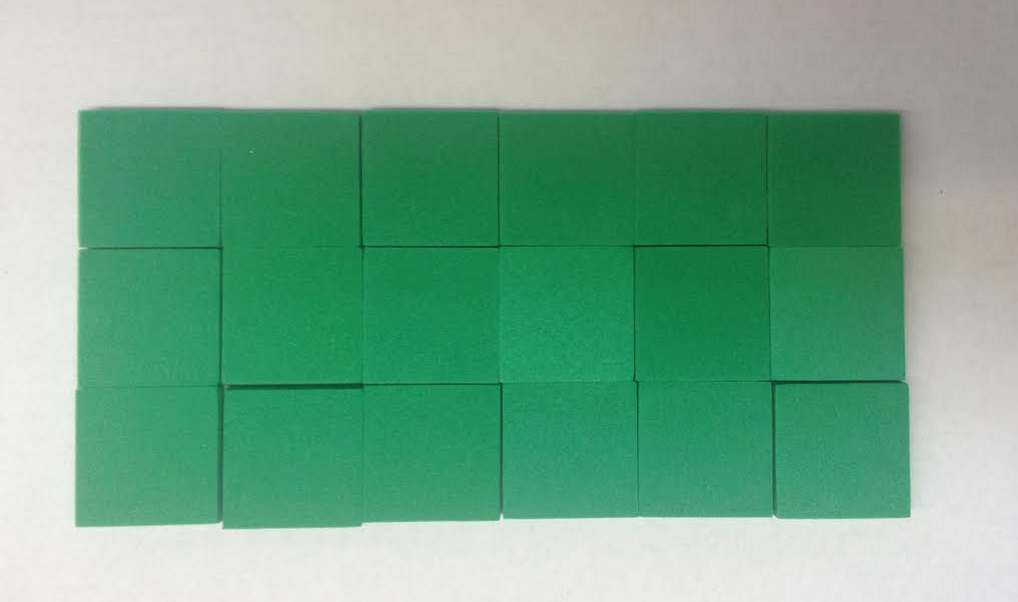
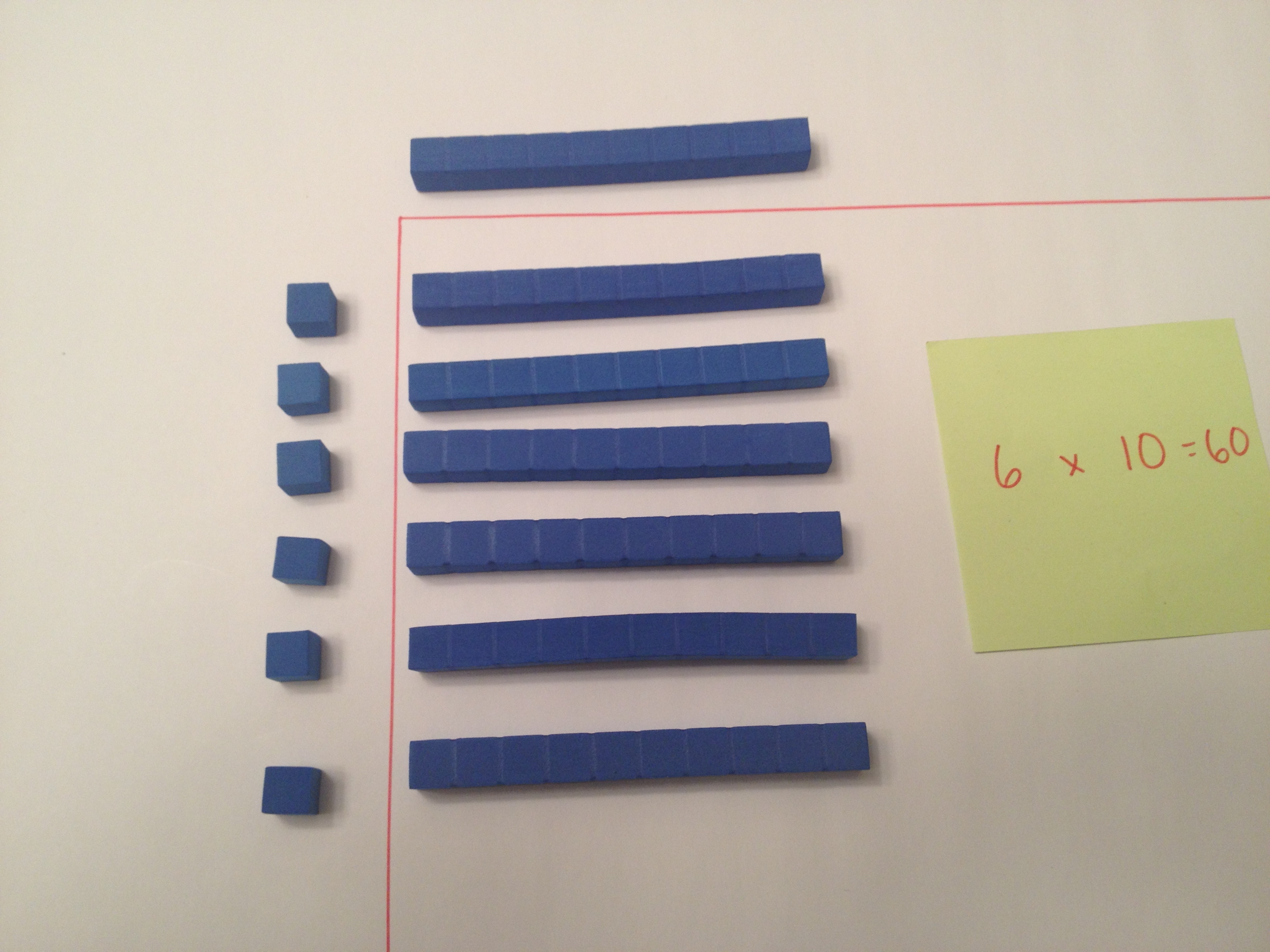
The model above is typically described as 3 x 6 but it could also represent division by giving students 18 tiles and have them construct a rectangle with 3 rows. The 18 tiles now become the dividend (not the product), which takes flexibility in understanding.
What happens if you ask students to build a model with 19 tiles and place them in 3 rows?
19 can be divided into three rows but the context will determine what to do with the 1 remaining tile. The blue tile can be recognized as a remainder, as a third, or as 1/6 of the next group. Using “untidy” number combinations can open the door to some awesome discussion.
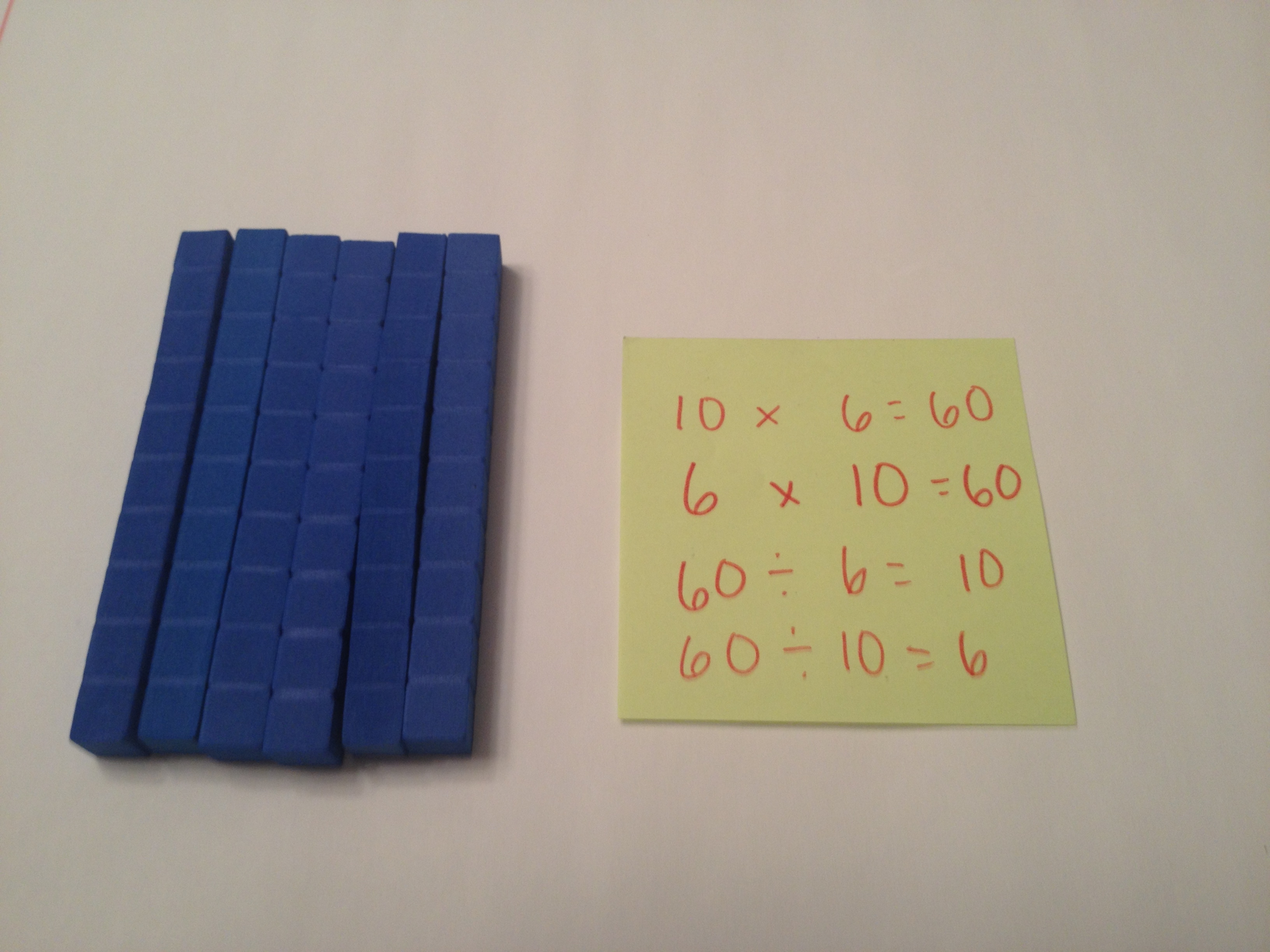
Third grade students also begin to multiply with single-digit factors and multiples of 10.
- 3.NBT.3 – Multiply one-digit whole numbers by multiples of 10 in the range of 10-90 using strategies based on place value and properties of operations.
If multiplication and division are taught concurrently, then students begin to understand that the model below has multiple meanings but it depends on the context. Flexibility is really hard to teach. So don’t teach it…provide opportunities that foster flexible thinking.
The difficult thing for students to recognize ties back to an understanding that is built in kindergarten…unitizing. Even though these are “ten rods”, students need to see them as a rod composed of ten ones. If students only see them as rods of 10 it becomes extremely difficult to see this model as division.
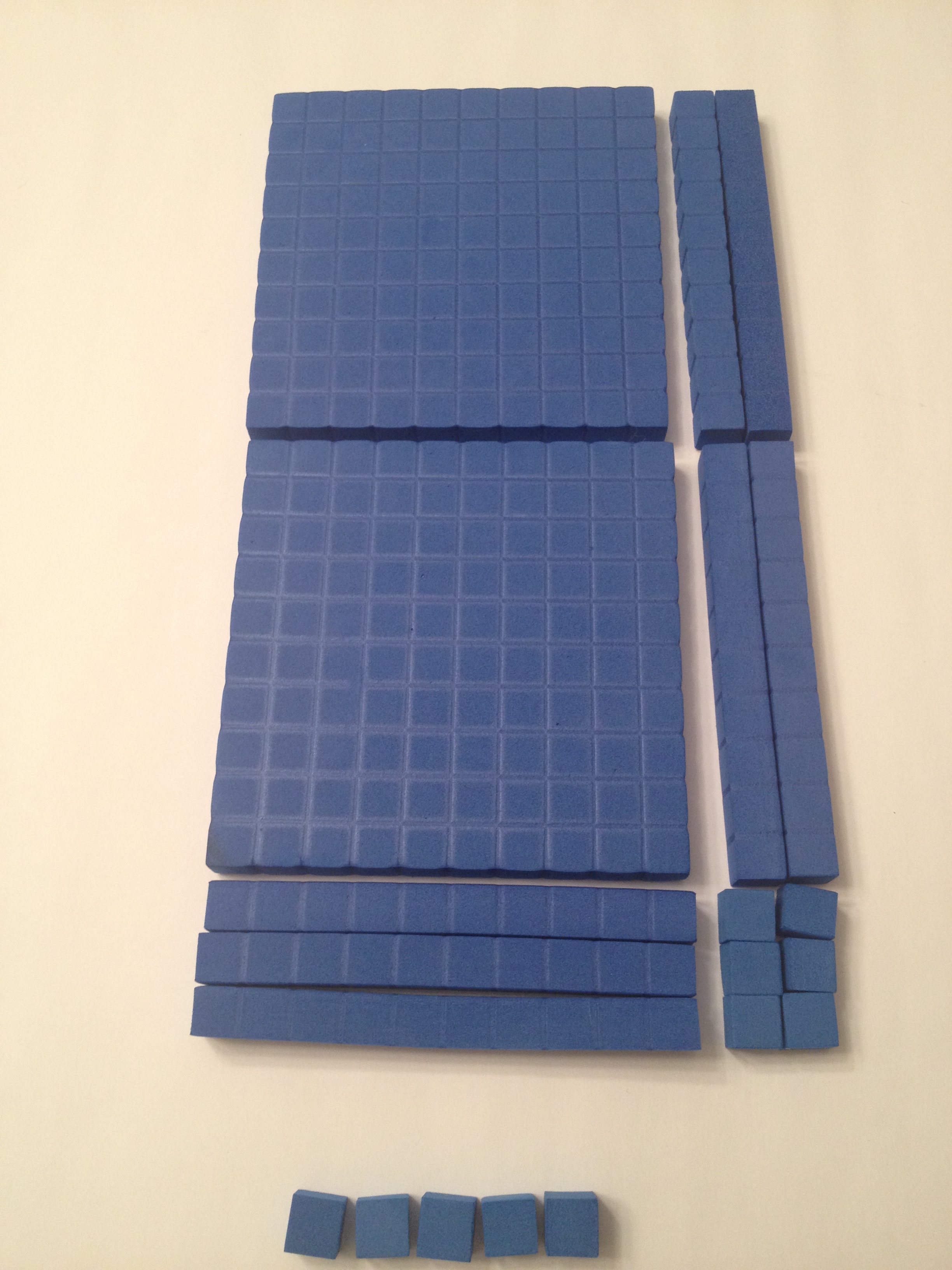
4th Grade: students begin to explore multiplication and division with multi-digit numbers.
Grade 5
- 5.NBT.5 – Fluently multiply multi-digit whole numbers using the standard algorithm
- 5.NBT.6 – Find whole-number quotients of whole numbers with up to four-digit dividends and two-digit divisors
So back to our original picture…if the outside of the area model is viewed as quotient and divisor (not factors), and area model itself is viewed as the dividend, the 5 “leftover” squares have meaning.
Here’s how I teach area model for division with students and teachers…

This is delicious, Graham, right down to the dessert at the end. This post was the highlight of a dinner table discussion last night with Paul and Phil, which revolved around how context in learning is so critical to engagement regardless of the content. As Paul said, “The story is everything.” Your removal of context reveals whether there is understanding of all the bare bones model can represent, while subtly reiterating how important context is to specific interpretation and application. Favorite quote: “Flexibility is really hard to teach. So don’t teach it…provide opportunities that foster flexible thinking.” Thanks for the opportunity.
I wish I could be a fly on the wall when you all dive into these conversations. Hearing that some of the smartest people I know (ie: engineers) stress the importance of teaching in context tells me we’re doing the right work.
Thanks for allowing me to virtually sit at your table to feed my brain.
Thanks for such a meaty post! I have an untested theory that we learn lots of things “forward”, but the usefulness might often be more in the “backward” (adding and subtracting, multiplying and dividing or factoring, derivatives and integrals, etc.).
Thanks for the comment Belinda and I couldn’t agree more with the idea of learning backwards.
I think a strong case can be made for either forward or backwards learning. Kind of like the chicken or the egg idea of which goes first. I think it’s different for individual students however I think most teachers will agree that there needs to be a healthy dose of both..